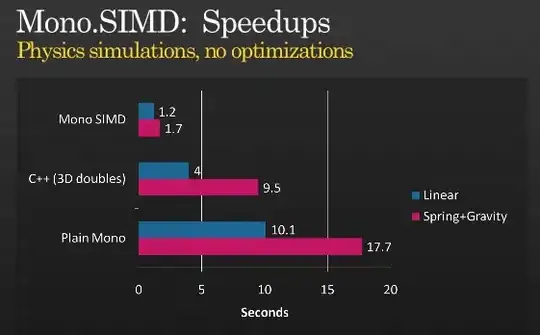
Given the following numpy matrix
import numpy as np
np_matrix = np.array(
[[0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,3,0,0,0,3,0,2,0,0,1,0,0,0,0,0,0]
,[0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,3,0,0,0,3,0,2,2,0,0,0,0,0,0,0,0]
,[0,0,0,3,0,0,0,0,2,2,2,0,0,0,0,0,3,0,0,0,3,0,0,2,2,2,2,2,2,2,2,2]
,[0,0,0,3,0,0,0,2,0,0,0,2,0,0,0,0,3,0,0,0,3,3,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,3,0,0,2,0,1,0,0,0,2,0,0,0,3,0,0,0,0,3,3,3,3,3,0,0,0,0,0,0]
,[0,0,0,3,0,0,2,0,0,0,0,0,2,0,0,0,3,0,0,0,0,0,0,0,0,3,3,3,3,3,3,3]
,[0,0,0,3,0,0,2,0,0,0,0,0,2,0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,0,3,0,0,2,0,0,0,2,0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,0,3,0,0,0,2,2,2,0,0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,0,0,3,0,0,0,0,0,0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,0,0,0,3,0,0,0,0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0]
,[3,3,3,3,0,0,0,3,0,0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,3,0,0,0,0,3,3,3,3,0,0,0,0,0,3,3,3,3,0,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,0,3,0,0,0,0,0,0,0,0,0,0,3,3,3,0,0,3,3,3,3,0,0,0,0,0,0,0,0]
,[0,0,0,0,3,3,0,0,0,0,0,0,0,0,0,3,0,0,0,0,0,0,0,3,0,0,0,0,0,0,0,0]
,[0,0,0,0,0,3,0,0,0,0,0,0,0,0,0,3,0,0,2,2,2,0,0,3,0,0,0,0,0,0,0,0]
,[2,2,2,0,0,3,0,0,0,0,0,0,0,0,0,3,0,0,2,1,2,0,0,3,0,0,0,0,0,0,0,0]
,[0,0,2,2,0,3,3,0,0,0,0,0,0,0,0,3,3,0,2,2,2,0,0,3,0,0,0,0,0,0,0,0]
,[0,0,0,2,0,0,3,0,0,0,0,0,0,0,0,0,3,0,0,0,0,0,0,3,0,0,0,0,0,0,0,0]
,[1,0,0,2,0,0,3,0,0,0,0,0,0,0,0,0,3,3,0,0,0,0,3,0,0,0,0,0,0,0,0,0]
,[0,0,0,2,0,0,3,0,0,0,0,0,0,0,0,0,0,3,3,0,3,3,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,2,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,3,3,0,0,0,0,0,0,0,0,0,0,0]
,[0,0,2,2,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0]
,[2,2,2,0,0,0,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0]
,[0,0,0,0,0,3,3,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,3,3,3,3,3,3,3,3]
,[0,0,0,0,0,3,0,0,0,0,0,0,3,3,3,3,3,3,3,3,0,0,0,0,3,0,0,0,0,0,0,0]
,[0,0,0,3,3,0,0,0,0,3,3,3,3,2,2,2,2,2,2,3,3,0,0,0,3,0,0,0,0,0,2,2]
,[3,3,3,3,0,0,0,0,0,3,2,2,2,0,0,0,0,0,2,2,3,0,0,0,3,0,0,0,2,2,2,0]
,[0,0,0,0,0,0,0,0,0,3,2,0,0,0,0,0,0,0,0,2,3,0,0,0,3,0,0,2,2,0,0,0]
,[0,0,0,0,0,0,0,0,0,3,2,0,0,0,1,0,0,0,0,2,3,0,0,0,3,0,0,2,0,0,0,1]]
)
Which can be presented visually in a picture like this:

Where the red dots are numbered from left to right and can be identified in the matrix using the following function. Thanks to @DanielF in this answer
def getRedDotsCoordinatesFromLeftToRight(np_matrix, red_dor_number=1):
red_dots = np.where(np_matrix == red_dor_number)
red_dots = tuple(g[np.argsort(red_dots[-1])] for g in red_dots)
red_dots = np.stack(red_dots)[::-1].T
return red_dots
red_dots = getRedDotsCoordinatesFromLeftToRight(np_matrix)
print(red_dots)
red_dots = np.array(
[[ 0, 25],
[ 4, 8],
[16, 19],
[19, 0],
[29, 14],
[29, 31]]
)
Two questions:
- Question 1: How can we identify all the white points coordinates (marked with
0) within the green boundaries (marked with2) which are located with red dots (marked with1) ? - Question 2: How can we identify all the white points coordinates (marked with
0) between black boundaries (marked with3) and the green boundaries (marked with2) which are located with red dots (marked with1) ?
I am looking for this result for this example matrix:
space_within_greenDots = np.array(
[[[17, 0], [17, 1], [18, 0], [18, 1], [18, 2], [19, 1], [19, 2], [20, 0], [20, 1], [20, 2], [21, 0], [21, 1], [21, 2], [22, 0], [22, 1]],
[[ 3, 8], [ 3, 9], [ 3, 10], [ 4, 7], [ 4, 9], [ 4, 10], [ 4, 11], [ 5, 7], [ 5, 8], [ 5, 9], [ 5, 10], [ 5, 11], [ 6, 7], [ 6, 8], [ 6, 9], [ 6, 10], [ 6, 11], [ 7, 8], [ 7, 9], [ 7, 10]],
[[27, 13], [27, 14], [27, 15], [27, 16], [27, 17], [28, 11], [28, 12], [28, 13], [28, 14], [28, 15], [28, 16], [28, 17], [28, 18], [29, 11], [29, 12], [29, 13], [29, 15], [29, 16], [29, 17], [29, 18]],
[],
[[ 0, 23], [ 0, 24], [ 0, 26], [ 0, 27], [ 0, 28], [ 0, 29], [ 0, 30], [ 0, 31], [ 1, 24], [ 1, 25], [ 1, 26], [ 1, 27], [ 1, 28], [ 1, 29], [ 1, 30], [ 1, 31]],
[[27, 31], [28, 29], [28, 30], [28, 31], [29, 28], [29, 29], [29, 30]]],
)
space_between_darkDots_and_greenDots = np.array(
[ [[12, 0], [12, 1], [12, 2], [13, 0], [13, 1], [13, 2], [13, 3], [14, 0], [14, 1], [14, 2], [14, 3], [15, 0], [15, 1], [15, 2], [15, 3], [15, 4], [16, 3], [16, 4], [17, 4], [18, 4], [18, 5], [19, 4], [19, 5], [20, 4], [20, 5], [21, 4], [21, 5], [22, 4], [22, 5], [23, 3], [23, 4], [23, 5], [24, 0], [24, 1], [24, 2], [24, 3], [24, 4], [25, 0], [25, 1], [25, 2], [25, 3], [25, 4], [26, 0], [26, 1], [26, 2]],
[[ 0, 4], [ 0, 5], [ 0, 6], [ 0, 7], [ 0, 8], [ 0, 9], [ 0, 10], [ 0, 11], [ 0, 12], [ 0, 13], [ 0, 14], [ 0, 15], [ 1, 4], [ 1, 5], [ 1, 6], [ 1, 7], [ 1, 8], [ 1, 9], [ 1, 10], [ 1, 11], [ 1, 12], [ 1, 13], [ 1, 14], [ 1, 15], [ 2, 4], [ 2, 5], [ 2, 6], [ 2, 7], [ 2, 11], [ 2, 12], [ 2, 13], [ 2, 14], [ 2, 15], [ 3, 4], [ 3, 5], [ 3, 6], [ 3, 12], [ 3, 13], [ 3, 14], [ 3, 15], [ 4, 4], [ 4, 5], [ 4, 13], [ 4, 14], [ 4, 15], [ 5, 4], [ 5, 5], [ 5, 13], [ 5, 14], [ 5, 15], [ 6, 4], [ 6, 5], [ 6, 13], [ 6, 14], [ 6, 15], [ 7, 5], [ 7, 6], [ 7, 12], [ 7, 13], [ 7, 14], [ 8, 5], [ 8, 6], [ 8, 7], [ 8, 11], [ 8, 12], [ 8, 13], [ 8, 14], [ 9, 6], [ 9, 7], [ 9, 8], [ 9, 9], [ 9, 10], [ 9, 11], [ 9, 12], [ 9, 13], [10, 7], [10, 8], [10, 9], [10, 10], [10, 11], [10, 12], [11, 8], [11, 9], [11, 10], [11, 11]],
[],
[[13, 18], [13, 19], [14, 16], [14, 17], [14, 18], [14, 19], [14, 20], [14, 21], [14, 22], [15, 16], [15, 17], [15, 21], [15, 22], [16, 16], [16, 17], [16, 21], [16, 22], [17, 17], [17, 21], [17, 22], [18, 17], [18, 18], [18, 19], [18, 20], [18, 21], [18, 22], [19, 18], [19, 19], [19, 20], [19, 21], [20, 19]],
[[ 0, 21], [ 1, 21], [ 2, 21], [ 2, 22], [ 3, 22], [ 3, 23], [ 3, 24], [ 3, 25], [ 3, 26], [ 3, 27], [ 3, 28], [ 3, 29], [ 3, 30], [ 3, 31], [ 4, 26], [ 4, 27], [ 4, 28], [ 4, 29], [ 4, 30], [ 4, 31]],
[[25, 25], [25, 26], [25, 27], [25, 28], [25, 29], [25, 30], [25, 31], [26, 25], [26, 26], [26, 27], [26, 28], [26, 29], [27, 25], [27, 26], [27, 27], [28, 25], [28, 26], [29, 25], [29, 26]],
]
)
A few assumptions:
- The matrix shape can vary. It is not a fixed size.
- The number of red dots varies from matrix to matrix. But there is always at least one red dot in the matrix.¨