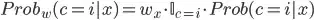The paper about SAMME.R algorithm
Firstly,in the 2a step, if fits a classifier T(x) to the training data using weights,but I don't know how the algorithm uses the classifier T(x) in the following part.
Secondly, in the 2b step, i don't know how to obtain the weighted class probability estimates. It just says we can use decision tree to estimate the probability, but I don't know how to do it.
Thanks in advance. My English is poor and my question may be vague. I am really sorry for this. If you can't understand my question, just comment it and I will try my best to expound my question clearly! Thank you very much!!