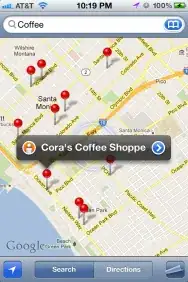
How may I take a shape that was created with more points at its curves and subdivide it so that the points are distributed more equally along the curve? In my research I thought that numpy's interp might be the right function to use, but I don't know what to use for the parameters (x, xp, fp, left, right, & period). Any help would be very appreciated!
Here is an animation showing the desired output.
This is the code for the input rounded rectangle:
from matplotlib import pyplot as plt
import numpy as np
x_values = [1321.4, 598.6, 580.6, 563.8, 548.6, 535.4, 524.5, 516.2, 511,
509.2, 509.2, 511, 516.2, 524.5, 535.4, 548.6, 563.8, 580.6, 598.6, 1321.4, 1339.4,
1356.2, 1371.4, 1384.6, 1395.5, 1403.8, 1409, 1410.8, 1410.8, 1409, 1403.8, 1395.5,
1384.6, 1371.4, 1356.2, 1339.4, 1321.4]
y_values = [805.4, 805.4, 803.5, 798.3, 790.1,
779.2, 766, 750.8, 734, 716, 364, 346, 329.2, 314, 300.8, 289.9, 281.7, 276.5, 274.6,
274.6, 276.5, 281.7, 289.9, 300.8, 314, 329.2, 346, 364, 716, 734, 750.8, 766, 779.2,
790.1, 798.3, 803.5, 805.4]
fig, ax = plt.subplots(1)
ax.plot(x_values,y_values)
ax.scatter(x_values,y_values)
ax.set_aspect('equal')
plt.show()
Thank you!