The R heatmap() documentation says for Rowv and Colv (i.e. row and column ordering parameters):
If either is missing, as by default, then the ordering of the corresponding dendrogram is by the mean value of the rows/columns, i.e., in the case of rows, Rowv <- rowMeans(x, na.rm = na.rm).
I thought it's as easy as that but now I guess there must be something more in the default ordering algorithm.
Let's have this correlation matrix:
m = matrix(nrow=7, ncol = 7, c(1,0.578090870728824,0.504272263365781,0.526539138953634,0.523049273011785,0.503296777916728,0.638770769734758,0.578090870728824,1,0.59985543029105,0.663649941610205,0.630998114483389,0.66814547270115,0.596161809036262,0.504272263365781,0.59985543029105,1,0.62468477053142,0.632715952452297,0.599037620726669,0.607925540860012,0.526539138953634,0.663649941610205,0.62468477053142,1,0.7100707346884,0.738094117424525,0.639668277558577,0.523049273011785,0.630998114483389,0.632715952452297,0.7100707346884,1,0.651331659193182,0.64138213322125,0.503296777916728,0.66814547270115,0.599037620726669,0.738094117424525,0.651331659193182,1,0.612326706593738,0.638770769734758,0.596161809036262,0.607925540860012,0.639668277558577,0.64138213322125,0.612326706593738,1))
m
[,1] [,2] [,3] [,4] [,5] [,6] [,7]
[1,] 1.0000000 0.5780909 0.5042723 0.5265391 0.5230493 0.5032968 0.6387708
[2,] 0.5780909 1.0000000 0.5998554 0.6636499 0.6309981 0.6681455 0.5961618
[3,] 0.5042723 0.5998554 1.0000000 0.6246848 0.6327160 0.5990376 0.6079255
[4,] 0.5265391 0.6636499 0.6246848 1.0000000 0.7100707 0.7380941 0.6396683
[5,] 0.5230493 0.6309981 0.6327160 0.7100707 1.0000000 0.6513317 0.6413821
[6,] 0.5032968 0.6681455 0.5990376 0.7380941 0.6513317 1.0000000 0.6123267
[7,] 0.6387708 0.5961618 0.6079255 0.6396683 0.6413821 0.6123267 1.0000000
The heatmap(m) output is:
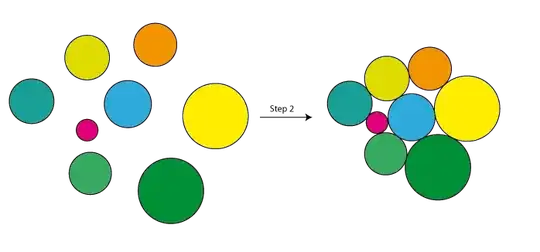
The row (and column) order is: 1, 3, 7, 5, 2, 6, 4
However, I expected the ordering to be:
order(rowMeans(m))
1 3 7 2 6 5 4
How's that?
I guess it could have something to do with how the dendrograms are clustered. But still unsure: if I first group 4 and 6 and then perhaps work with a 6x6 matrix where one row/column is the averages(?) of the original rows 4 and 6, it still shouldn't change the mutual order of e.g. rows 2 and 5, should it?
Thank you very much for any hint!