Is there any real practical difference between a SortedList<TKey,TValue> and a SortedDictionary<TKey,TValue>? Are there any circumstances where you would specifically use one and not the other?
- 36,951
- 69
- 249
- 387
-
Related: [When to use a SortedList or a SortedDictionary](http://stackoverflow.com/questions/1376965/when-to-use-a-sortedlisttkey-tvalue-over-a-sorteddictionarytkey-tvalue) – Liam Oct 29 '14 at 08:13
-
19I'm confused. Why does SortedList have two type parameters `SortedList
` rather than one `SortedList – Colonel Panic Jul 27 '15 at 13:38`? Why doesn't it implement `IList `? -
3@ColonelPanic because functionally SortedList is a map, not a linear collection. Dont let the name fool you. Just like a dictionary, you pass in a key, you get a value back. While dictionary is unordered, SortedList is ordered in its natural sorted order. – nawfal Jul 31 '15 at 18:30
7 Answers
Yes - their performance characteristics differ significantly. It would probably be better to call them SortedList and SortedTree as that reflects the implementation more closely.
Look at the MSDN docs for each of them (SortedList, SortedDictionary) for details of the performance for different operations in different situtations. Here's a nice summary (from the SortedDictionary docs):
The
SortedDictionary<TKey, TValue>generic class is a binary search tree with O(log n) retrieval, where n is the number of elements in the dictionary. In this, it is similar to theSortedList<TKey, TValue>generic class. The two classes have similar object models, and both have O(log n) retrieval. Where the two classes differ is in memory use and speed of insertion and removal:
SortedList<TKey, TValue>uses less memory thanSortedDictionary<TKey, TValue>.
SortedDictionary<TKey, TValue>has faster insertion and removal operations for unsorted data, O(log n) as opposed to O(n) forSortedList<TKey, TValue>.If the list is populated all at once from sorted data,
SortedList<TKey, TValue>is faster thanSortedDictionary<TKey, TValue>.
(SortedList actually maintains a sorted array, rather than using a tree. It still uses binary search to find elements.)
- 1,421,763
- 867
- 9,128
- 9,194
-
Thanks v much to all for the pointers. I guess I'm just too lazy to RTFM... much easier to ask the nice folks on SO... ;) I voted you both up for the answers; Jon gets answer credit for being first on the trigger. :) – Shaul Behr Jun 01 '09 at 16:56
-
2I think the SortedList definition should be corrected as I don't believe it's a binary search tree ... ? – nchaud Feb 05 '13 at 19:38
-
1I looked using reflector and found that it didn't use a binary search tree. – Daniel Imms Feb 23 '13 at 05:54
-
I think the Sorteddictionary is an AVL-tree or Red-Blacktree( all operation cost O(logn). And the SortedList is a binary-search (it costs o(n) time in the worst case)l – Ghoster Aug 15 '19 at 03:52
Here is a tabular view if it helps...
From a performance perspective:
+------------------+---------+----------+--------+----------+----------+---------+
| Collection | Indexed | Keyed | Value | Addition | Removal | Memory |
| | lookup | lookup | lookup | | | |
+------------------+---------+----------+--------+----------+----------+---------+
| SortedList | O(1) | O(log n) | O(n) | O(n)* | O(n) | Lesser |
| SortedDictionary | O(n)** | O(log n) | O(n) | O(log n) | O(log n) | Greater |
+------------------+---------+----------+--------+----------+----------+---------+
* Insertion is O(log n) for data that are already in sort order, so that each
element is added to the end of the list. If a resize is required, that element
takes O(n) time, but inserting n elements is still amortized O(n log n).
list.
** Available through enumeration, e.g. Enumerable.ElementAt.
From an implementation perspective:
+------------+---------------+----------+------------+------------+------------------+
| Underlying | Lookup | Ordering | Contiguous | Data | Exposes Key & |
| structure | strategy | | storage | access | Value collection |
+------------+---------------+----------+------------+------------+------------------+
| 2 arrays | Binary search | Sorted | Yes | Key, Index | Yes |
| BST | Binary search | Sorted | No | Key | Yes |
+------------+---------------+----------+------------+------------+------------------+
To roughly paraphrase, if you require raw performance SortedDictionary could be a better choice. If you require lesser memory overhead and indexed retrieval SortedList fits better. See this question for more on when to use which.
- 70,104
- 56
- 326
- 368
-
Note that if you want good performance **and** relatively low memory usage **and** indexed retrieval, consider [`BDictionary
` in LoycCore](http://loyc.net/doc/code/classLoyc_1_1Collections_1_1BDictionary_3_01K_00_01V_01_4.html) instead of `SortedDictionary`. – Qwertie Feb 26 '16 at 06:13 -
1Yes, look at the bottom part of [this article](http://core.loyc.net/collections/alists-part3.html). It turns out `BDictionary` is usually slower than `SortedDictionary` except for very large sizes, but it is faster than `SortedList` if there are over 700 items or so. Memory use should be only slightly higher than `SortedList` (much lower than `SortedDictionary`), due to the use of arrays in the leaves of the tree. – Qwertie Mar 15 '16 at 03:08
-
1It's unfortunate that ordered insertion into SortedList is O(log n) per element. Making it O(1) would have been easy to implement with just one check before performing a binary search. – relatively_random Nov 06 '20 at 17:10
I cracked open Reflector to have a look at this as there seems to be a bit of confusion about SortedList. It is in fact not a binary search tree, it is a sorted (by key) array of key-value pairs. There is also a TKey[] keys variable which is sorted in sync with the key-value pairs and used to binary search.
Here is some source (targeting .NET 4.5) to backup my claims.
Private members
// Fields
private const int _defaultCapacity = 4;
private int _size;
[NonSerialized]
private object _syncRoot;
private IComparer<TKey> comparer;
private static TKey[] emptyKeys;
private static TValue[] emptyValues;
private KeyList<TKey, TValue> keyList;
private TKey[] keys;
private const int MaxArrayLength = 0x7fefffff;
private ValueList<TKey, TValue> valueList;
private TValue[] values;
private int version;
SortedList.ctor(IDictionary, IComparer)
public SortedList(IDictionary<TKey, TValue> dictionary, IComparer<TKey> comparer) : this((dictionary != null) ? dictionary.Count : 0, comparer)
{
if (dictionary == null)
{
ThrowHelper.ThrowArgumentNullException(ExceptionArgument.dictionary);
}
dictionary.Keys.CopyTo(this.keys, 0);
dictionary.Values.CopyTo(this.values, 0);
Array.Sort<TKey, TValue>(this.keys, this.values, comparer);
this._size = dictionary.Count;
}
SortedList.Add(TKey, TValue) : void
public void Add(TKey key, TValue value)
{
if (key == null)
{
ThrowHelper.ThrowArgumentNullException(ExceptionArgument.key);
}
int num = Array.BinarySearch<TKey>(this.keys, 0, this._size, key, this.comparer);
if (num >= 0)
{
ThrowHelper.ThrowArgumentException(ExceptionResource.Argument_AddingDuplicate);
}
this.Insert(~num, key, value);
}
SortedList.RemoveAt(int) : void
public void RemoveAt(int index)
{
if ((index < 0) || (index >= this._size))
{
ThrowHelper.ThrowArgumentOutOfRangeException(ExceptionArgument.index, ExceptionResource.ArgumentOutOfRange_Index);
}
this._size--;
if (index < this._size)
{
Array.Copy(this.keys, index + 1, this.keys, index, this._size - index);
Array.Copy(this.values, index + 1, this.values, index, this._size - index);
}
this.keys[this._size] = default(TKey);
this.values[this._size] = default(TValue);
this.version++;
}
- 47,944
- 19
- 150
- 166
Enough is said already on the topic, however to keep it simple, here's my take.
Sorted dictionary should be used when-
- More inserts and delete operations are required.
- Data in un-ordered.
- Key access is enough and index access is not required.
- Memory is not a bottleneck.
On the other side, Sorted List should be used when-
- More lookups and less inserts and delete operations are required.
- Data is already sorted (if not all, most).
- Index access is required.
- Memory is an overhead.
Hope this helps!!
- 469
- 6
- 12
This is visual representation of how performances compare to each other.
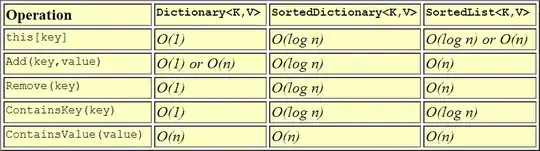
- 3,719
- 6
- 41
- 56
-
1Where did you take that info from? From this scheme we can see that Dictinary is better in any way, so there is no reason for others to exist. – Cute pumpkin Jan 29 '19 at 11:18
-
1@alexkostin Maybe a bit late but see https://stackoverflow.com/a/19702706/7224691. I was having issues with the source link but i could find it on the wayback machine. – Ben Jan 09 '21 at 03:15
-
1@alexkostin `Dictionary
` does not preserve the insertion order of its elements, where as `SortedList – Steve Guidi Feb 04 '22 at 03:37` and `SortedDictionary ` order by a given `IComparer `.
Check out the MSDN page for SortedList:
From Remarks section:
The
SortedList<(Of <(TKey, TValue>)>)generic class is a binary search tree withO(log n)retrieval, wherenis the number of elements in the dictionary. In this, it is similar to theSortedDictionary<(Of <(TKey, TValue>)>)generic class. The two classes have similar object models, and both haveO(log n)retrieval. Where the two classes differ is in memory use and speed of insertion and removal:
SortedList<(Of <(TKey, TValue>)>)uses less memory thanSortedDictionary<(Of <(TKey, TValue>)>).
SortedDictionary<(Of <(TKey, TValue>)>)has faster insertion and removal operations for unsorted data,O(log n)as opposed toO(n)forSortedList<(Of <(TKey, TValue>)>).If the list is populated all at once from sorted data,
SortedList<(Of <(TKey, TValue>)>)is faster thanSortedDictionary<(Of <(TKey, TValue>)>).
-
9The quoted text is wrong (and was updated on MSDN): SortedList is not a "binary search tree", it is an "array of key/value pairs". – Eldritch Conundrum Aug 19 '12 at 23:24
Index access (mentioned here) is the practical difference. If you need to access the successor or predecessor, you need SortedList. SortedDictionary cannot do that so you are fairly limited with how you can use the sorting (first / foreach).
- 1,232
- 10
- 21